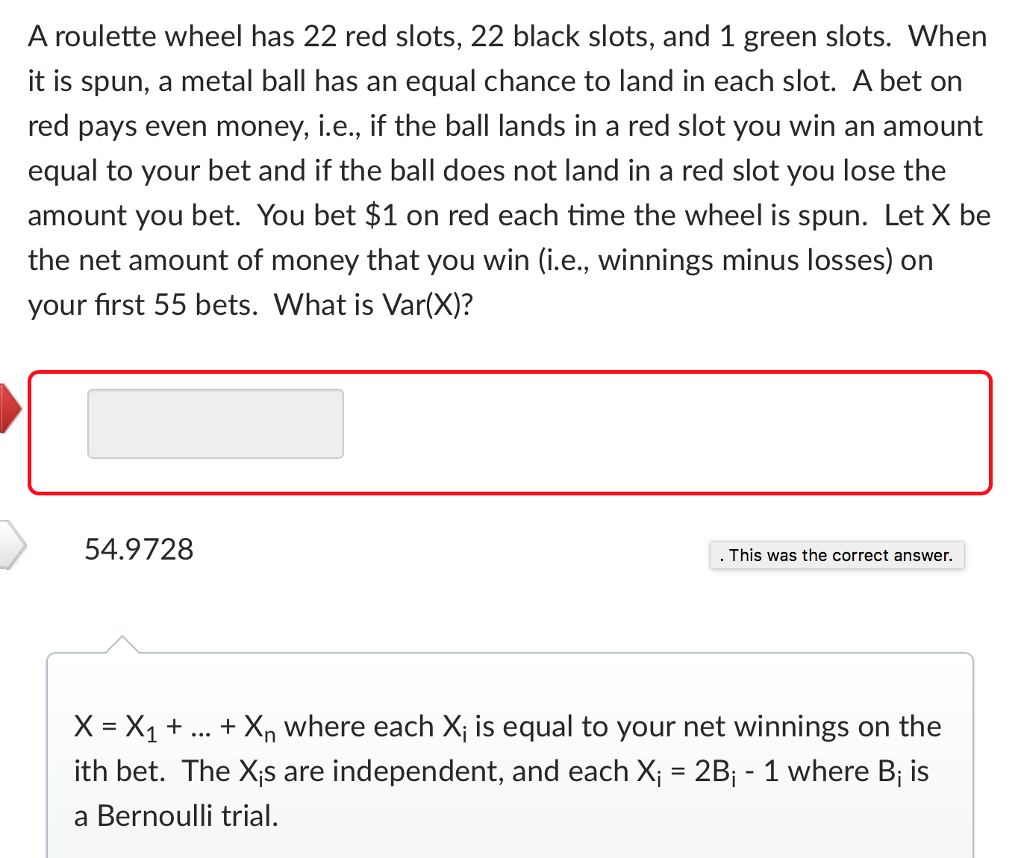
Roulette Bernoulli
A Bernoulli Distribution is the probability distribution of a random variable which takes the value 1 with probability p and value 0 with probability 1 – p, i.e.
$$
begin{cases}
1-p & text{for} k=0
p & text{for} k=1
end{cases}$$
We will use the example of left-handedness. Approximately 10% of the population are left-handed (p=0.1).
- Let us first consider the very simple situation where the fluid is static—that is, v 1 = v 2 = 0. Bernoulli’s equation in that case is. P 1 + ρgh 1 = P 2 + ρgh 2. We can further simplify the equation by taking h 2 = 0 (we can always choose some height to be zero, just as we often have done for other situations involving the gravitational force, and take all other heights to be relative.
- Details The Bernoulli distribution with prob = p has density p (x) = p x (1 − p) 1 − x for x = 0 o r 1. If an element of x is not 0 or 1, the result of dbern is zero, without a warning. P (x) is computed using Loader's algorithm, see the reference below.
We want to know, out of a random sample of 10 people, what is the probability of 3 of these 10 people being left handed?
We assign a 1 to each person if they are left handed and 0 otherwise:
- $P(X=1) = 0.1$
- $P(X=0) = 0.9$
A Binomial distribution is derived from the Bernoulli distribution.
Roulette Bernoulli Games
We’ll start with the simpler problem:
Bernoulli argued that people should be maximizing expected utility not expected value u( x) is the expected utility of an amount Moreover, marginal utility should be decreasing The value of an additional dollar gets lower the more money you have For example u($0) = 0 u($499,999) = 10 u($1,000,000) = 16.
Roulette Bernoulli Experiment
What is the probability of the first 3 people we pick being left-handed, followed by 7 people being right-handed?
This is just $ 0.1 ^3 times 0.9 ^7$
What if we wanted the last 3 people to be left-handed?
This is just $0.9^7 times 0.1^3$, the same answer.
In fact, no matter how we arrange the 3 people, we will always end up with the same probability ($ 4.7 times 10^{-4} $).
So we have to add up all the ways we can arrange the 3 people being picked.
Bernoulli Roulette System
There are $10!$ ways to arrange 10 people and there are $3!$ ways to arrange the 3 people that are picked and $7!$ ways to arrange the 7 people that aren’t picked.
This is given as:
$$dfrac{10!}{3! 7!}$$

Or more commonly, “10 choose 3”. The “n choose k” notation is written as:
$$
begin{equation*}
binom{n}{k}
end{equation*} = dfrac{n!}{k! (n-k)!}
$$
We can now caclulate the probability that there are 3 left-handed people in a random selection of 10 people as:
$$
P(X=3) = begin{equation*}
binom{10}{3}
end{equation*} (0.1)^3 (0.9)^7
$$
This will generalise such that:

$$
P(X=k) = begin{equation*}
binom{n}{k}
end{equation*} (p)^k (1-p)^{n-k}
$$
Scipy’s stats package has a binomial package that can be used to calculate these probabilities:
We can use this function to calculate what the probability of 3 or fewer people being left-handed from a selection of 10 people.
$$
P(X leq 3) = sum_{i=0}^{3} begin{equation*}
binom{10}{i}
end{equation*} (0.1)^i (0.9)^{n-i}
$$
Or we could plot our probability results for each value up to all 10 people being left-handed:
We can see there is almost negligible chance of getting more than 6 left-handed people in a random group of 10 people.
Roulette
On an American roulette wheel there are 38 squares:
- 18 black
- 18 red
- 2 green

We bet on black 10 times in a row, what are the chances of winning more than half of these?
$$
P(X gt 5) = sum_{i=6}^{10} begin{equation*}
binom{10}{i}
end{equation*} bigg(dfrac{18}{38}bigg)^i bigg(1-dfrac{18}{38}bigg)^{n-i}
$$
/close-up-of-roulette-wheel-1059428626-0bfd3dca97294b6f915f791deece0ef4.jpg)
A Poisson distribution is a limiting version of the binomial distribution, where $n$ becomes large and $np$ approaches some value $lambda$, which is the mean value.
The Poisson distribution can be used for the number of events in other specified intervals such as distance, area or volume. Examples that may follow a Poisson include the number of phone calls received by a call center per hour and the number of decay events per second from a radioactive source.
It is calculated as:
$ P(k) = e^{-lambda} dfrac{lambda^k}{k!} $
The average number of goals in a World Cup football match is 2.5.
We would like to know the probability of 4 goals in a match.
Again, scipy has in-built functions for calculating this and we can use this to calculate the probability of any number of goals in a World Cup match.